Q r factorization calculator

Related calculator: LU Decomposition Calculator. The QR Factorization Calculator is a resource for efficient and precise matrix factorization. Our digital tool excels in delivering step-by-step QR decomposition. Input Input the elements of the matrix you want to factorize in the provided fields, q r factorization calculator.

We use cookies to improve your experience on our site and to show you relevant advertising. By browsing this website, you agree to our use of cookies. Learn more. Matrix operations Method 1. Transforming matrix to Row Echelon Form 2. Transforming matrix to Reduced Row Echelon Form 3.

Q r factorization calculator

Welcome to Omni's QR decomposition calculator! Here you can determine the QR decompositions of square and rectangular matrices. Not sure what the QR decomposition or QR factorization is? We will give you the definition of the QR decomposition for square matrices and tell you how it extends to rectangular matrices with more rows than columns. We will explain how to find the QR decomposition and we will discuss the main application of the QR factorization, that is, how it can facilitate solving systems of linear equations. At the very end there will be, of course, a detailed step-by-step example of QR decomposition. Let's go! Decomposition or factorization of a matrix is the process of representing this matrix as a product of two or more matrices that have various special properties. The idea is that various matrix operations become simpler thanks to the special properties of constituent matrices. There are lots of different types of matrix decompositions; among the most popular types there is the QR decomposition, which is the topic of this page, as well as the LU decomposition , Cholesky decomposition , and the singular value decomposition SVD. In the QR decomposition, we factor a real square matrix A of size n x n into the product of two matrices:. If A is invertible and we require the diagonal entries of R to be positive, then the QR decomposition is unique.

Eigenvectors 7. Our digital tool excels in delivering step-by-step QR decomposition. Click on the "Calculate" button.

.

Welcome to Omni's QR decomposition calculator! Here you can determine the QR decompositions of square and rectangular matrices. Not sure what the QR decomposition or QR factorization is? We will give you the definition of the QR decomposition for square matrices and tell you how it extends to rectangular matrices with more rows than columns. We will explain how to find the QR decomposition and we will discuss the main application of the QR factorization, that is, how it can facilitate solving systems of linear equations. At the very end there will be, of course, a detailed step-by-step example of QR decomposition.

Q r factorization calculator

Related calculator: LU Decomposition Calculator. The QR Factorization Calculator is a resource for efficient and precise matrix factorization. Our digital tool excels in delivering step-by-step QR decomposition. Input Input the elements of the matrix you want to factorize in the provided fields. The matrix can be either square or rectangular. Click on the "Calculate" button. You can use these matrices for further computations or analysis as needed. Mathematically, it is represented as. This decomposition technique is important in several mathematical operations, such as solving linear equations, matrix inversion, and calculating eigenvalues. The QR factorization of a matrix is not always unique.

Black queen bedspread

Matrix size. We will explain how to find the QR decomposition and we will discuss the main application of the QR factorization, that is, how it can facilitate solving systems of linear equations. Eigenvectors 7. Eigenvalues 6. How to find the QR decomposition? Power Method for dominant eigenvalue Click the advanced mode button and adjust the precision field according to your needs. Method 1. Let's go! Pivots Let's focus on R. In consequence, the left-hand side simplifies as follows:. The penultimate equation is now also simple to solve as it involves only v and z and we already know the value of z!

.

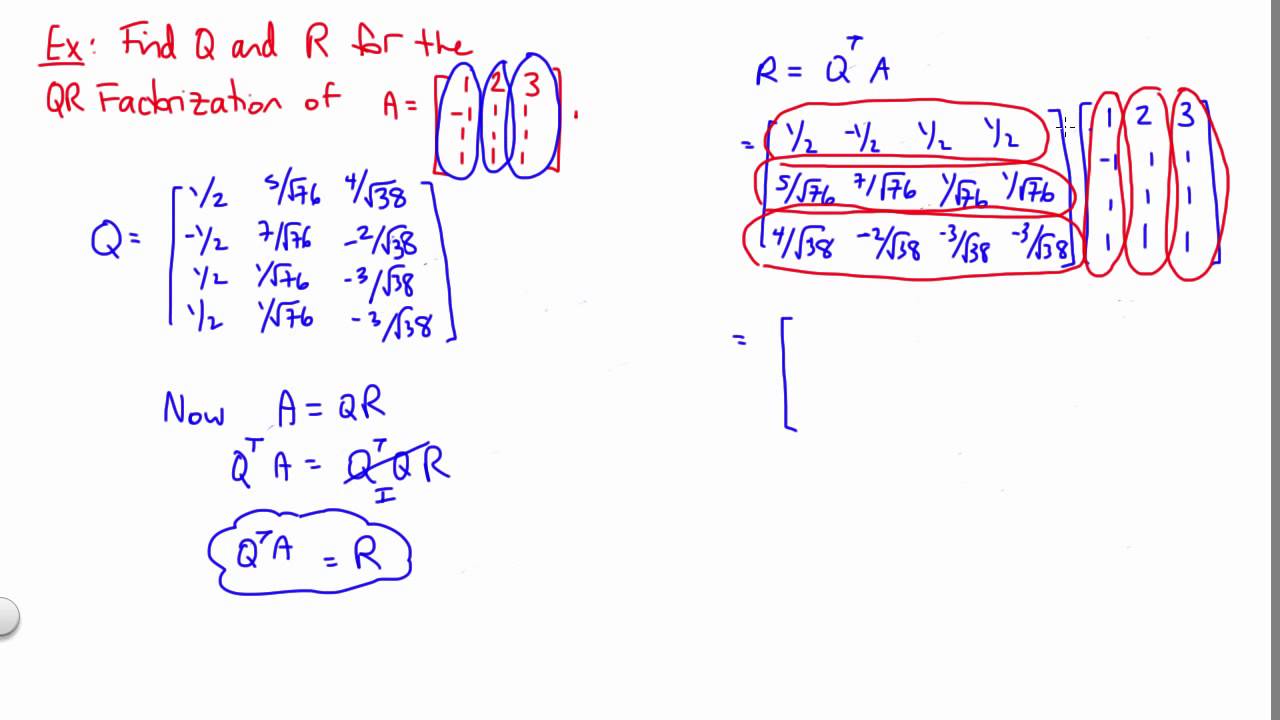
How accurate is the QR Factorization Calculator? Eigenvalues 6. Without such constraints, QR factorization is not unique. Pseudoinverse Formula 1. Does a QR decomposition always exist? In such a case, the QR decomposition is not unique. In the QR decomposition, we factor a real square matrix A of size n x n into the product of two matrices:. Matrix size. Another important field where QR decomposition is often used is in calculating the eigenvalues and eigenvectors of a matrix. Yes, every square matrix and even rectangular matrix has a QR decomposition. Equivalent Fractions Calculator. There are several methods for deriving the QR decomposition of a matrix: Gram—Schmidt orthogonalization - this method is easy to understand even with only basic knowledge of linear algebra, but it's numerically unstable and so not very useful in real-life applications. Equivalently, you can determine R by left-multiplying the original matrix A by the transpose of our newly-found matrix Q as follows:.