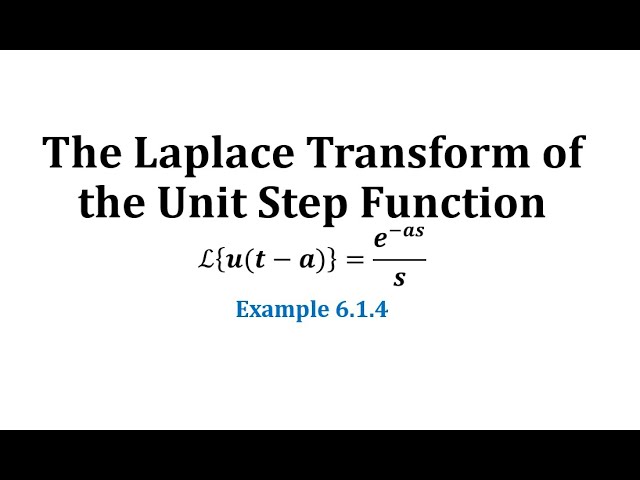
Laplace transform of unit step function

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser.

Online Calculus Solver ». IntMath f orum ». In engineering applications, we frequently encounter functions whose values change abruptly at specified values of time t. One common example is when a voltage is switched on or off in an electrical circuit at a specified value of time t. The switching process can be described mathematically by the function called the Unit Step Function otherwise known as the Heaviside function after Oliver Heaviside. That is, u is a function of time t , and u has value zero when time is negative before we flip the switch ; and value one when time is positive from when we flip the switch. In this work, it doesn't make a great deal of difference to our calculations, so we'll continue to use the first interpretation, and draw our graphs accordingly.

Laplace transform of unit step function

To productively use the Laplace Transform, we need to be able to transform functions from the time domain to the Laplace domain. We can do this by applying the definition of the Laplace Transform. Our goal is to avoid having to evaluate the integral by finding the Laplace Transform of many useful functions and compiling them in a table. Thereafter the Laplace Transform of functions can almost always be looked by using the tables without any need to integrate. A table of Laplace Transform of functions is available here. In this case we say that the "region of convergence" of the Laplace Transform is the right half of the s-plane since s is a complex number, the right half of the plane corresponds to the real part of s being positive. As long as the functions we are working with have at least part of their region of convergence in common which will be true in the types of problems we consider , the region of convergence holds no particular interest for us. Since the region of convergence will not play a part in any of the problems we will solve, it is not considered further. The unit impulse is discussed elsewhere , but to review. The area of the impulse function is one.

That is why, when choosing the basic functions that make up the composite function, only addition is allowed. Posted 9 years ago. So how could I construct this function right here using my unit step function?

Online Calculus Solver ». IntMath f orum ». We saw some of the following properties in the Table of Laplace Transforms. We write the function using the rectangular pulse formula. We also use the linearity property since there are 2 items in our function. This is an exponential function see Graphs of Exponential Functions.

Online Calculus Solver ». IntMath f orum ». We saw some of the following properties in the Table of Laplace Transforms. We write the function using the rectangular pulse formula. We also use the linearity property since there are 2 items in our function. This is an exponential function see Graphs of Exponential Functions.

Laplace transform of unit step function

Online Calculus Solver ». IntMath f orum ». In engineering applications, we frequently encounter functions whose values change abruptly at specified values of time t. One common example is when a voltage is switched on or off in an electrical circuit at a specified value of time t. The switching process can be described mathematically by the function called the Unit Step Function otherwise known as the Heaviside function after Oliver Heaviside. That is, u is a function of time t , and u has value zero when time is negative before we flip the switch ; and value one when time is positive from when we flip the switch. In this work, it doesn't make a great deal of difference to our calculations, so we'll continue to use the first interpretation, and draw our graphs accordingly.

Ronaldo boxershorts

So what's going to happen? Transform of Unit Step Functions. In the next video, we'll do a bunch of examples where we can apply this, but we should at least prove to ourselves what the Laplace transform of this thing is. So in this case, it's the Laplace transform of sine of t. It is the function f that is varying. It stays at zero until some value. Email Address Sign Up. And also, if we took the derivative of both sides of this, or I guess the differential, you would get dx is equal to dt. Let me write that down. Comment Button navigates to signup page. Let me write that. Table of Laplace Transformations 3. Transform of Periodic Functions 6. Let me draw some function.

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser.

So now that we had this, let's go back and make that substitution that x is equal to t minus c. Well, let's say we wanted to figure out the Laplace transform of the unit step function that starts up at pi of t. So what if I-- my new function, I call it the unit step function up until c of t times f of t minus c? Rather that for use with the Laplace transform, we are only interested in c values greater than zero since, as you mentioned, the transform is only concerned with t [0,inf. It's zero. I keep getting stuck around 20 min where Sal explains that x is just a letter. Let's say it's at 2 until I get to pi. That was the big takeaway from this video. Let's say it goes to zero until-- I don't know, I'll call that c again. So our integral becomes-- I'll do it in green-- when t is equal to c, what is x? Example: Laplace Transform of a Gated Sine Find the Laplace Transform of the function shown: Solution : This function is a little different than the previous in that it involves more than ramps and steps. We could go in this direction. Functionally we want: so Graphically this is shown as: Aside: An improper method to solve the problem It may seem like it would be easier to define the function as a ramp 0.