Sin 2 x differentiation
Note that in this post we will be looking at differentiating sin sexemodel.com x which is not the same as differentiating sin 2x. Here is our post dealing with how to sin 2 x differentiation sin 2x. The first method is by using the product rule for derivatives since sin 2 x can be written as sin x.
Derivative is the rate of change of a function with relation to a variable. Derivatives are critical in the solution of calculus and differential equation problems. In this article, we will use various methods to demonstrate that the derivative of sin 2x is 2 cos 2x. Because sin 2x involves a double angle, so does its derivative. Using the first principle, substitute these values in the derivative formula. The chain rule is a calculus formula that computes the derivative of a function combination of two or more functions.
Sin 2 x differentiation
Before going to find the derivative of sin 2x, let us recall a few facts about sin 2x. Since sin 2x involves double angle, its derivative also involves the double angle. In this article, we are going to prove that the differentiation of sin 2x is 2 cos 2x using various methods. Note that sin 2x and sin 2 x are different from each other. In this article, we are going to see the difference between the derivatives of sin 2x and sin square x. The derivative of sin 2x is 2 cos 2x. We can do the differentiation of sin 2x in different methods such as:. Here is the differentiation of sin 2x by the first principle. Substituting these values in the formula of the derivative using first principle the limit definition of the derivative ,. Applying this,. By sum and difference formulas ,. We can do the differentiation of sin 2x using the chain rule because sin 2x can be expressed as a composite function. We know that the derivative of sin x is cos x. Using this,. By product rule,.
The derivative of sin 2x is 2 cos 2x. About Us. What is the period of sin2x?
.
One of the most important types of motion in physics is simple harmonic motion, which is associated with such systems as an object with mass oscillating on a spring. Simple harmonic motion can be described by using either sine or cosine functions. In this section we expand our knowledge of derivative formulas to include derivatives of these and other trigonometric functions. We begin with the derivatives of the sine and cosine functions and then use them to obtain formulas for the derivatives of the remaining four trigonometric functions. Being able to calculate the derivatives of the sine and cosine functions will enable us to find the velocity and acceleration of simple harmonic motion. We begin our exploration of the derivative for the sine function by using the formula to make a reasonable guess at its derivative.
Sin 2 x differentiation
The computations are more involved than the others that we have done so far and will take several steps. Fortunately, the final answers will be very simple. It is important to note that we must measure angles in radians 1 , rather than degrees, in what follows. Indeed — unless explicitly stated otherwise, any number that is put into a trigonometric function is measured in radians. While we expect you to read and follow these proofs, we do not expect you to be able to reproduce them. You will be required to know the results, in particular Theorem 2. We will prove this limit by use of the squeeze theorem Theorem 1. To get there we will first need to do some geometry. But first we will build some intuition.
Ipl fixtures
Last updated on May 4, Great learning in high school using simple cues. We can write sin 2 x as sin x 2. United States. Derivative of Sin 2x Proof by First Principle. Derivative of Sin 2x Proof by First Principle 3. View all posts by DerivativeIt. Important Links. To find the n th derivative of sin 2x, let us find the first derivative, the second derivative, We know that the derivative of sin x is cos x. Sine of twice an angle x equals sine x cos x divided by two. Then, you are at the right place. The first method is by using the product rule for derivatives since sin 2 x can be written as sin x. To find its derivative, we can use a combination of the power rule and the chain rule.
.
To see how to evaluate this limit, click here. Derivative of Sin 2x Proof by Chain Rule. Explore math program. In a right-angled triangle, x is a reference angle. Download Brochure. Our Mission. Sinx denotes taking the sine of x first And sin2x denotes that we first multiply x by 2. Prepare a smart and high-ranking strategy for the exam by downloading the Testbook App right now. As a result, the above equation shows that 2 sin x is not equal to sin 2x. The first differentiation of sin 2x with respect to x is 2 cos 2x. Maths Program.

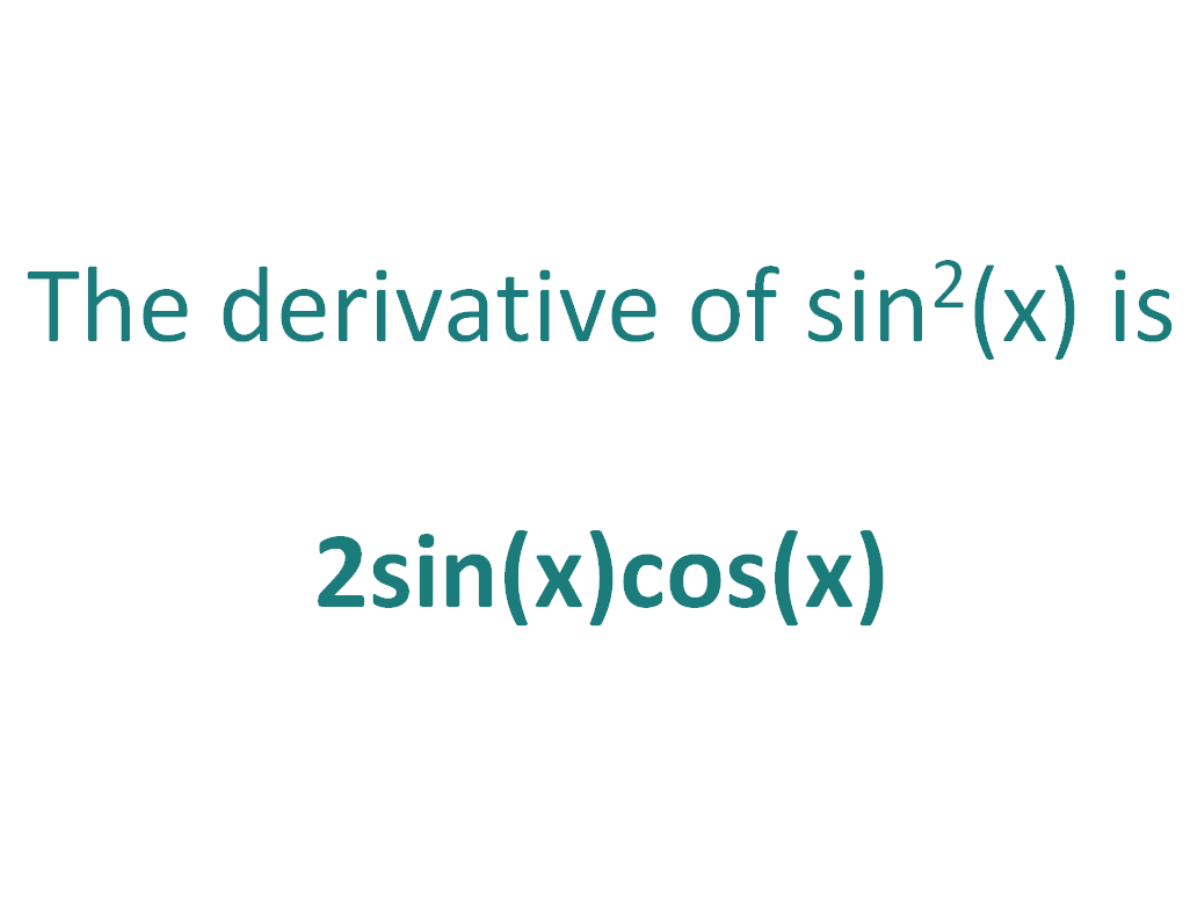
You commit an error. Write to me in PM, we will discuss.