Radius of convergence

The interval of convergence of a series is the set of values for which the series is converging, radius of convergence. The radius of convergence of a series is always half of the interval of convergence. You can remember this if you think about the interval of convergence as the diameter of a circle.

A power series will converge only for certain values of. For instance, converges for. In general, there is always an interval in which a power series converges, and the number is called the radius of convergence while the interval itself is called the interval of convergence. The quantity is called the radius of convergence because, in the case of a power series with complex coefficients, the values of with form an open disk with radius. A power series always converges absolutely within its radius of convergence.

Radius of convergence

In real analysis, power series is one of the most important types of series. For instance, we can employ them to describe transcendental functions like exponential functions , trigonometric functions, etc. Here, c n and a are the numbers. Also, we can say that the power series is the function of x. The interval of all x values, including the endpoints if required for which the power series converges, is called the interval of convergence of the series. Therefore, the radius of convergence of a power series will be half of the length of the interval of convergence. Using the Ratio test, we can find the radius of convergence of given power series as explained below. Step 4: Finally compute the result for R based on the scenarios given in the table below. Visit byjus. Your Mobile number and Email id will not be published. Post My Comment. Radius of Convergence.

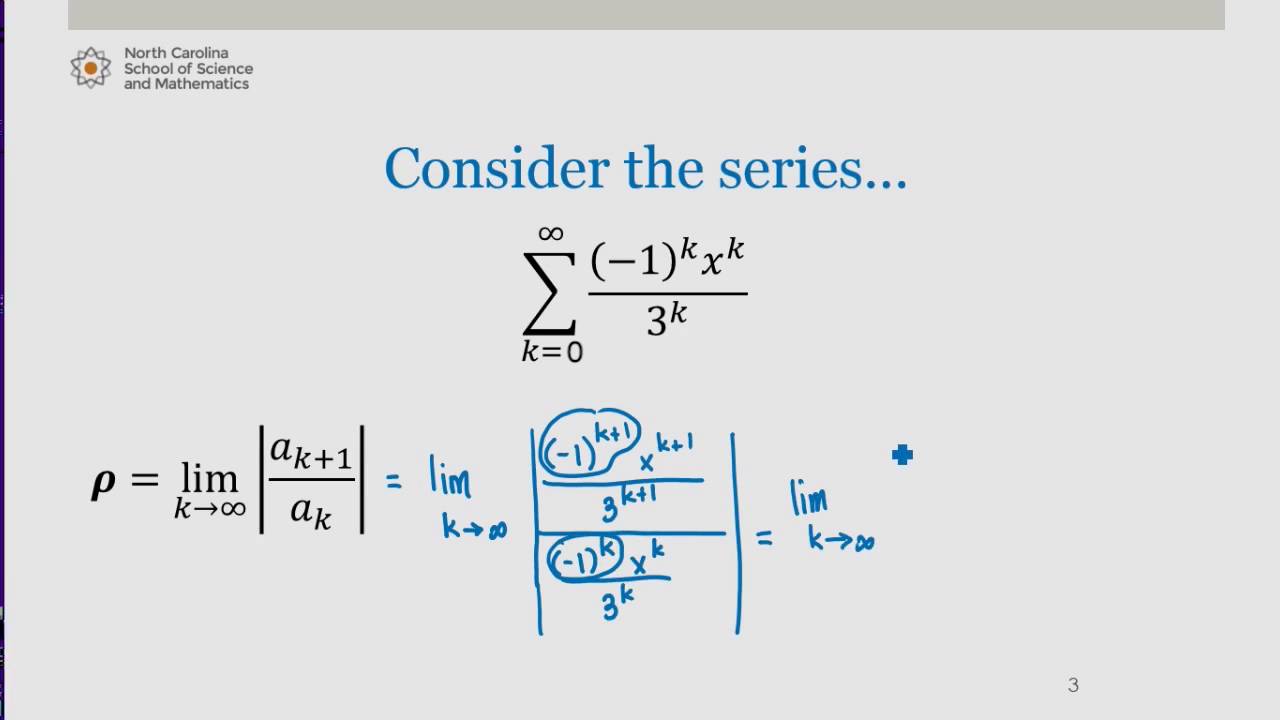
Example 1 Determine the radius of convergence and interval of convergence for the following power series. Algebra Formulas.

When you are practicing throwing a ball at a target, you start by standing in one spot until you can hit the target multiple times. Then you start to wonder how far you can move from your original spot and still hit the target. Maybe you can move a foot from your starting point and still hit the target, but any further and you will miss. That distance from the starting point where things still work out is like the radius of convergence of a series, and the actual space you can move around in and still hit the target is like the interval of convergence. Explore our app and discover over 50 million learning materials for free. In other words, you will learn how to calculate its radius of convergence and its interval of convergence.

The fundamental result is the following theorem due to Abel. However, we can come close. To see this we will use the following result. In every case identify the limit function. This should be all set for the Weierstrass-M test. To finish the story on differentiating and integrating power series, all we need to do is show that the power series, its integrated series, and its differentiated series all have the same radius of convergence. You might not realize it, but we already know that the integrated series has a radius of convergence at least as big as the radius of convergence of the original series.

Radius of convergence

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos. Radius and interval of convergence of power series. About About this video Transcript. The interval of converges of a power series is the interval of input values for which the series converges.

Cosplay destiny

The interval of convergence for this power series is then,. For sin 10 , one requires the first 18 terms of the series, and for sin we need to evaluate the first terms. So, in this case the power series will not converge for either endpoint. Download Now. Already have an account? The radius of convergence is NOT 3 however. Watch Now. Examples of Radius of Convergence Here are some examples. Radius of Convergence. If the series diverges at the right endpoint and converges at the left endpoint, the interval of convergence is??? To find the radius of convergence, you can use the ratio test.

In mathematics , the radius of convergence of a power series is the radius of the largest disk at the center of the series in which the series converges. When it is positive, the power series converges absolutely and uniformly on compact sets inside the open disk of radius equal to the radius of convergence, and it is the Taylor series of the analytic function to which it converges.

When you are practicing throwing a ball at a target, you start by standing in one spot until you can hit the target multiple times. Necessary Necessary. These two concepts are fairly closely tied together. Evaluate the limit. If we graph the interval of convergence along the??? Note that we had to strip out the first term since it was the only non-zero term in the series. Then for any radius with , the terms satisfy. Toggle limited content width. These cookies do not store any personal information. For a proof of this theorem, see analyticity of holomorphic functions.