Quadratic formula solver with steps
We often use this method when the leading coefficient is equal to 1 or If this is not the case, then it is better to use some other method.
Enter the coefficients that form a quadratic equation to solve for x using the quadratic formula. Joe is the creator of Inch Calculator and has over 20 years of experience in engineering and construction. He holds several degrees and certifications. Full bio. Ethan has a PhD in astrophysics and is currently a satellite imaging scientist. He specializes in math, science, and astrophysics. The quadratic formula can be used to calculate the solution for x in a quadratic equation.
Quadratic formula solver with steps
Solving equations is the central theme of algebra. All skills learned lead eventually to the ability to solve equations and simplify the solutions. In previous chapters we have solved equations of the first degree. You now have the necessary skills to solve equations of the second degree, which are known as quadratic equations. Upon completing this section you should be able to: Identify a quadratic equation. Place a quadratic equation in standard form. Solve a quadratic equation by factoring. A quadratic equation is a polynomial equation that contains the second degree, but no higher degree, of the variable. All quadratic equations can be put in standard form, and any equation that can be put in standard form is a quadratic equation. In other words, the standard form represents all quadratic equations. This theorem is proved in most college algebra books. An important theorem, which cannot be proved at the level of this text, states "Every polynomial equation of degree n has exactly n roots. It is possible that the two solutions are equal. A quadratic equation will have two solutions because it is of degree two.
The formula to calculate the axis of symmetry is:, quadratic formula solver with steps. The other term is either plus or minus two times the product of the square roots of the other two terms. While our first thought may be to try factoring, thinking about all the possibilities for trial and error method leads us to choose the Quadratic Formula as the most appropriate method.
If you missed this problem, review Figure. Simplify: If you missed this problem, review Figure. Solve Quadratic Equations Using the Quadratic Formula When we solved quadratic equations in the last section by completing the square, we took the same steps every time. Mathematicians look for patterns when they do things over and over in order to make their work easier. In this section we will derive and use a formula to find the solution of a quadratic equation. Now we will go through the steps of completing the square using the general form of a quadratic equation to solve a quadratic equation for x.
We often use this method when the leading coefficient is equal to 1 or If this is not the case, then it is better to use some other method. In this case, when the middle term is equal 0 we can use the difference of squares formula. This method solves all types of quadratic equations. It works best when solutions contain some radicals or complex numbers. Step 2 :Plug the values for a, b, and c into the quadratic formula and simplify.
Quadratic formula solver with steps
Instructions: This quadratic formula calculator will solve a quadratic equation for you, showing all the steps. Type the coefficients of the quadratic equation, and the solver will give you the roots, the y-intercept, the coordinates of the vertex showing all the work and it will plot the function. This is the main formula that determines a quadratic equation. Good news is that the above equation is not too hard to solve, which is a great thing considering that the quadratic equation appears literally everywhere in Algebra, Calculus and pretty much everywhere. Now, the question is how to solve this quadratic formula. Fortunately, the answer simple and well-known: It has solutions of the form. These are known as the roots of the quadratic equation also known as solutions of the equation. In order to analyze the nature of the solution, the discriminant is defined as:. Based on the value of the discriminant, the nature of the solutions is defined.
Maybelline colossal mascara review
Simple Interest. To solve a quadratic equation by completing the square, follow these steps: Step 1 If the coefficient of x 2 is not 1, divide all terms by that coefficient. Check: We leave the check for you! Learning Objectives By the end of this section, you will be able to: Solve quadratic equations using the Quadratic Formula Use the discriminant to predict the number and type of solutions of a quadratic equation Identify the most appropriate method to use to solve a quadratic equation. Now we will go through the steps of completing the square using the general form of a quadratic equation to solve a quadratic equation for x. Notice that once the radicand is simplified it becomes 0 , which leads to only one solution. Now let's consider how we can use completing the square to solve quadratic equations. Review Checkmark. The process of outlining and setting up the problem is the same as taught in chapter 5, but with problems solved by quadratics you must be very careful to check the solutions in the problem itself. What Is a Quadratic Equation? Distribute to get the equation in standard form. To use this theorem we put the equation in standard form, factor, and set each factor equal to zero. Methods for Solving Quadratic Equations. Enter a quadratic equation:.
Enter a math problem on an Equation in the text area Above.
This we did in the previous section many times. Isolate the variable terms on one side. When you encounter an incomplete quadratic with c - 0 third term missing , it can still be solved by factoring. So if we take the square root of -4, for example, we get:. In this example 6 and -1 are called the elements of the set. Result In a short moment, the calculator will show the roots. The method needed is called "completing the square. What Is a Quadratic Equation? We will solve the general quadratic equation by the method of completing the square. If we get a radical as a solution, the final answer must have the radical in its simplified form. Write the Quadratic Formula.

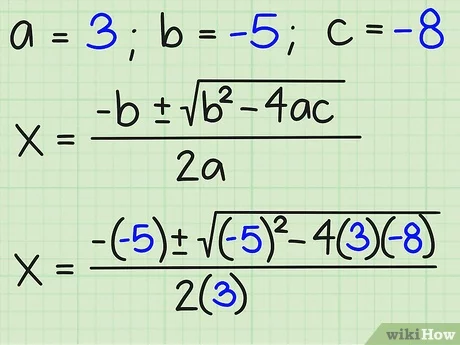
0 thoughts on “Quadratic formula solver with steps”