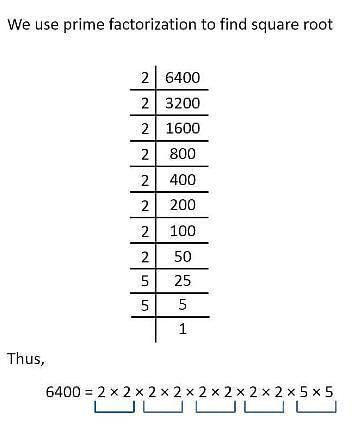
Prime factorization of 6400

The square root of is a number, which when multiplied by itself results in the number Here, we are going to discuss the value of the square root of and the methods such as prime factorization and the long division method to find the square root of are explained here in detail, prime factorization of 6400.

Let us first understand what is the square root of a number. The square root of a number is a value that can be multiplied by itself to give the original number. The prime factorization method is a way of finding the square root of a perfect square number. Note: It is a simple method and it is useful for large numbers that can be factored into primes or for small numbers who are perfect squares. This method works well with perfect square numbers.

Prime factorization of 6400

Factors of are the list of integers that we can split evenly into Factors of are pairs of those numbers whose products result in These factors are either prime numbers or composite numbers. To find the factors of , we will have to find the list of numbers that would divide without leaving any remainder. Further dividing 25 by 2 gives a non-zero remainder. So we stop the process and continue dividing the number 25 by the next smallest prime factor. We stop ultimately if the next prime factor doesn't exist or when we can't divide any further. Pair factors of are the pairs of numbers that when multiplied give the product The factors of in pairs are:. NOTE: If a, b is a pair factor of a number then b, a is also a pair factor of that number. The factors of are too many, therefore if we can find the prime factorization of , then the total number of factors can be calculated using the formula shown below. The factors of are 1, 2, 4, 5, 8, 10, 16, 20, 25, 32, 40, 50, 64, 80, , , , , , , , , , , , , and factors of are 1, 2, 4, , , Example 3: Find if 5, 16, 20, 25, 32, , and are factors of When we divide by it leaves a remainder.

Do you want a test? Or in other words, a number is a perfect square when it can be expressed as the product of an integer multiplied by itself. This is called the Product of Prime Factors of

Here we have a collection of all the information you may need about the Prime Factors of We will give you the definition of Prime Factors of , show you how to find the Prime Factors of Prime Factorization of by creating a Prime Factor Tree of , tell you how many Prime Factors of there are, and we will show you the Product of Prime Factors of Prime Factors of definition First note that prime numbers are all positive integers that can only be evenly divided by 1 and itself. Prime Factors of are all the prime numbers that when multiplied together equal To get the Prime Factors of , you divide by the smallest prime number possible.

You can also email us on info calculat. Prime Factorization of it is expressing as the product of prime factors. In other words it is finding which prime numbers should be multiplied together to make Since number is a Composite number not Prime we can do its Prime Factorization. To get a list of all Prime Factors of , we have to iteratively divide by the smallest prime number possible until the result equals 1. The smallest Prime Number which can divide without a remainder is 2. So the first calculation step would look like:. Now we have all the Prime Factors for number We may also express the prime factorization of as a Factor Tree :. Feedback form Hi!

Prime factorization of 6400

Factors of are the list of integers that we can split evenly into Factors of are pairs of those numbers whose products result in These factors are either prime numbers or composite numbers. To find the factors of , we will have to find the list of numbers that would divide without leaving any remainder. Further dividing 25 by 2 gives a non-zero remainder. So we stop the process and continue dividing the number 25 by the next smallest prime factor. We stop ultimately if the next prime factor doesn't exist or when we can't divide any further. Pair factors of are the pairs of numbers that when multiplied give the product The factors of in pairs are:.

Good hairdressers near me

Sri Lanka. The factors of are 1, 2, 4, 5, 8, 10, 16, 20, 25, 32, 40, 50, 64, 80, , , , , , , , , , , , , and its negative factors are -1, -2, -4, -5, -8, , , , , , , , , , , , , , , , , , , , , , Test Series. Square root of Download Brochure. United Kingdom. Saudi Arabia. Download as PDF. If you are checking Square Root of article, also check related maths articles:. Example 3: Find if 5, 16, 20, 25, 32, , and are factors of Let us first understand what is the square root of a number. Prime Factors of We hope this step-by-step tutorial to teach you about Prime Factors of was helpful. FREE Signup. Is the square root of a rational number? Report An Error.

Prime numbers are natural numbers positive whole numbers that sometimes include 0 in certain definitions that are greater than 1, that cannot be formed by multiplying two smaller numbers.

Maths Puzzles. All numbers except are factors of If so, try to find the Prime Factors of the next number on our list and then check your answer here. Factors of Solved Examples. Here we have a collection of all the information you may need about the Prime Factors of What is the value of the square root of ? Solution: The factors of are 1, 2, 4, 5, 8, 10, 16, 20, 25, 32, 40, 50, 64, 80, , , , , , , , , , , , , and factors of are 1, 2, 4, , , Example 3: Find if 5, 16, 20, 25, 32, , and are factors of Copyright Privacy Policy Disclaimer Contact. Hence, if we write the prime factorisation of in the radical form, it will not be in the simplest form. Do you want a test?