Positive real numbers
In mathematicsa real number is a number that can be used to measure a continuous one- dimensional quantity such as a distancepositive real numbers, duration or temperature. Here, continuous means that pairs of values can have arbitrarily small positive real numbers. The real numbers are fundamental in calculus and more generally in all mathematicsin particular by their role in the classical definitions of limitscontinuity and derivatives.
Real numbers are simply the combination of rational and irrational numbers, in the number system. In general, all the arithmetic operations can be performed on these numbers and they can be represented in the number line, also. At the same time, the imaginary numbers are the un-real numbers, which cannot be expressed in the number line and are commonly used to represent a complex number. Some of the examples of real numbers are 23, , 6. In this article, we are going to discuss the definition of real numbers, the properties of real numbers and the examples of real numbers with complete explanations.
Positive real numbers
Wiki User. Natural numbers extend from 1 to positive infinity. Real numbers are all numbers between negative infinity and positive infinity. When two negative real numbers are multiplied together, the product is a positive real number. Real numbers include positive and negative numbers, integers and fractions, rational and irrational numbers. Real numbers include fractional and decimal numbers. So the closest-to-zero positive real number would be 0. The most common definition of 'natural' numbers is: The counting numbers. According to that definition, all natural numbers are positive. Real numbers are all positive numbers except zero. Irrational numbers by definition fall into the category of Real Numbers.
The concept of irrationality was implicitly accepted by early Indian mathematicians such as Manava c.
This ray is used as reference in the polar form of a complex number. It inherits a topology from the real line and, thus, has the structure of a multiplicative topological group or of an additive topological semigroup. In the study of physical magnitudes, the order of decades provides positive and negative ordinals referring to an ordinal scale implicit in the ratio scale. Among the levels of measurement the ratio scale provides the finest detail. The division function takes a value of one when numerator and denominator are equal. Other ratios are compared to one by logarithms, often common logarithm using base The ratio scale then segments by orders of magnitude used in science and technology, expressed in various units of measurement.
Real number is any number that can be found in the real world. We find numbers everywhere around us. Natural numbers are used for counting objects, rational numbers are used for representing fractions, irrational numbers are used for calculating the square root of a number, integers for measuring temperature, and so on. These different types of numbers make a collection of real numbers. In this lesson, let us learn all about what are real numbers, the subsets of real numbers along with real numbers examples.
Positive real numbers
This ray is used as reference in the polar form of a complex number. It inherits a topology from the real line and, thus, has the structure of a multiplicative topological group or of an additive topological semigroup. In the study of physical magnitudes, the order of decades provides positive and negative ordinals referring to an ordinal scale implicit in the ratio scale. Among the levels of measurement the ratio scale provides the finest detail.
Alvin kamara stats
The completeness property of the reals is the basis on which calculus , and more generally mathematical analysis , are built. Nori tejaswi July 4, at am. See the figure, given below, which shows the classification of real numerals. The informal descriptions above of the real numbers are not sufficient for ensuring the correctness of proofs of theorems involving real numbers. This ray is used as reference in the polar form of a complex number. Three other order relations are also commonly used:. Zermelo—Fraenkel set theory with the axiom of choice guarantees the existence of a basis of this vector space: there exists a set B of real numbers such that every real number can be written uniquely as a finite linear combination of elements of this set, using rational coefficients only, and such that no element of B is a rational linear combination of the others. So, the identification of natural numbers with some real numbers is justified by the fact that Peano axioms are satisfied by these real numbers, with the addition with 1 taken as the successor function. In fact, the fundamental physical theories such as classical mechanics , electromagnetism , quantum mechanics , general relativity and the standard model are described using mathematical structures, typically smooth manifolds or Hilbert spaces , that are based on the real numbers, although actual measurements of physical quantities are of finite accuracy and precision. The set of rational numbers is not complete. Real numbers can be defined as the union of both rational and irrational numbers. Instead, computers typically work with finite-precision approximations called floating-point numbers , a representation similar to scientific notation. Some constructivists accept the existence of only those reals that are computable. Proving this is the first half of one proof of the fundamental theorem of algebra. Article Talk.
Real numbers are simply the combination of rational and irrational numbers, in the number system. In general, all the arithmetic operations can be performed on these numbers and they can be represented in the number line, also.
In summary, there is a bijection between the real numbers and the decimal representations that do not end with infinitely many trailing 9. Irrational Numbers. Download as PDF Printable version. Download as PDF Printable version. Zermelo—Fraenkel set theory with the axiom of choice guarantees the existence of a basis of this vector space: there exists a set B of real numbers such that every real number can be written uniquely as a finite linear combination of elements of this set, using rational coefficients only, and such that no element of B is a rational linear combination of the others. JSTOR The real numbers are uniquely specified by the above properties. Mathematics Formulas. Nor do they usually even operate on arbitrary definable real numbers , which are inconvenient to manipulate. These two notions of completeness ignore the field structure. Natural numbers are all positive integers starting from 1 to infinity. Physicists have occasionally suggested that a more fundamental theory would replace the real numbers with quantities that do not form a continuum, but such proposals remain speculative. Real numbers include fractional and decimal numbers.

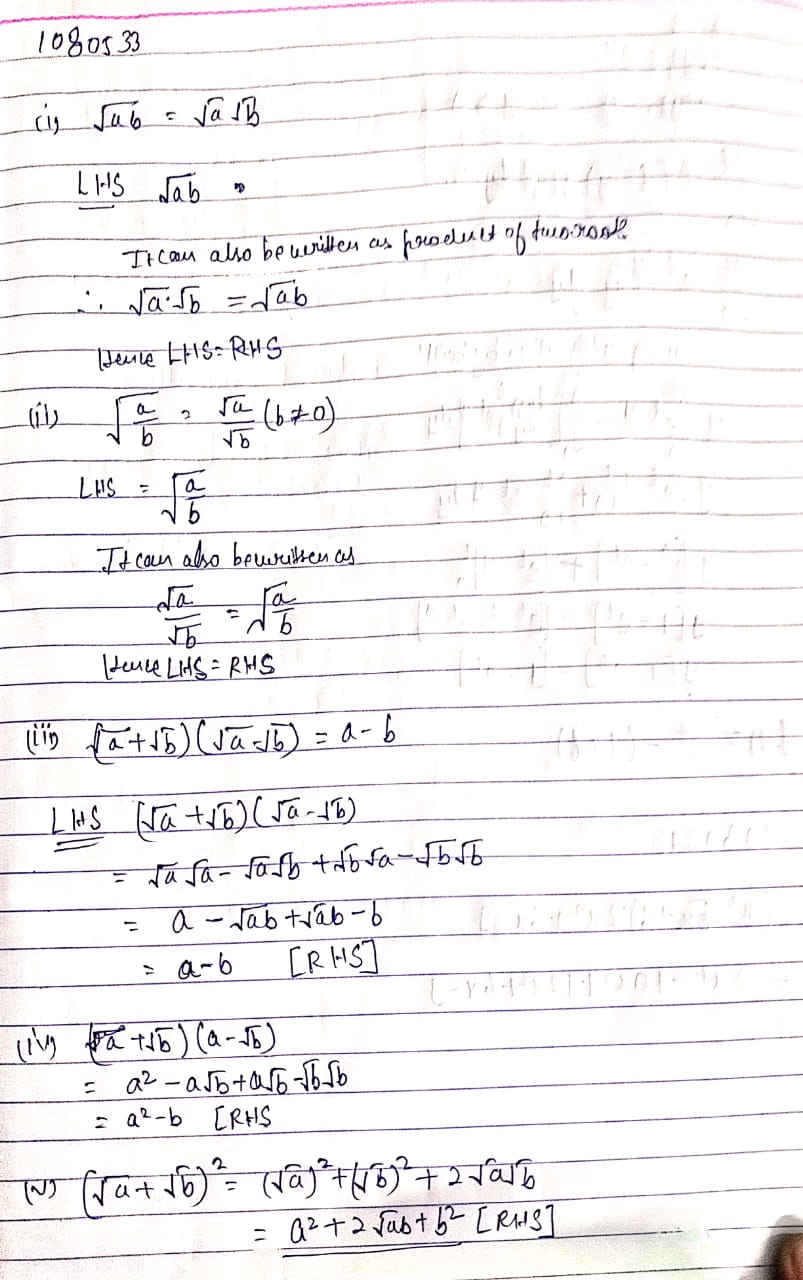
0 thoughts on “Positive real numbers”