Lcm with prime factorization

The word factor can be both a noun and a verb. To factor a number is to rewrite it by breaking it up into a product of smaller numbers. We say that 6 and 4 are factors of But so are 2 and

For two integers a and b, denoted LCM a,b , the LCM is the smallest positive integer that is evenly divisible by both a and b. The LCM of two or more numbers is the smallest number that is evenly divisible by all numbers in the set. Find the LCM of a set of numbers with this calculator which also shows the steps and how to do the work. Input the numbers you want to find the LCM for. You can use commas or spaces to separate your numbers.

Lcm with prime factorization

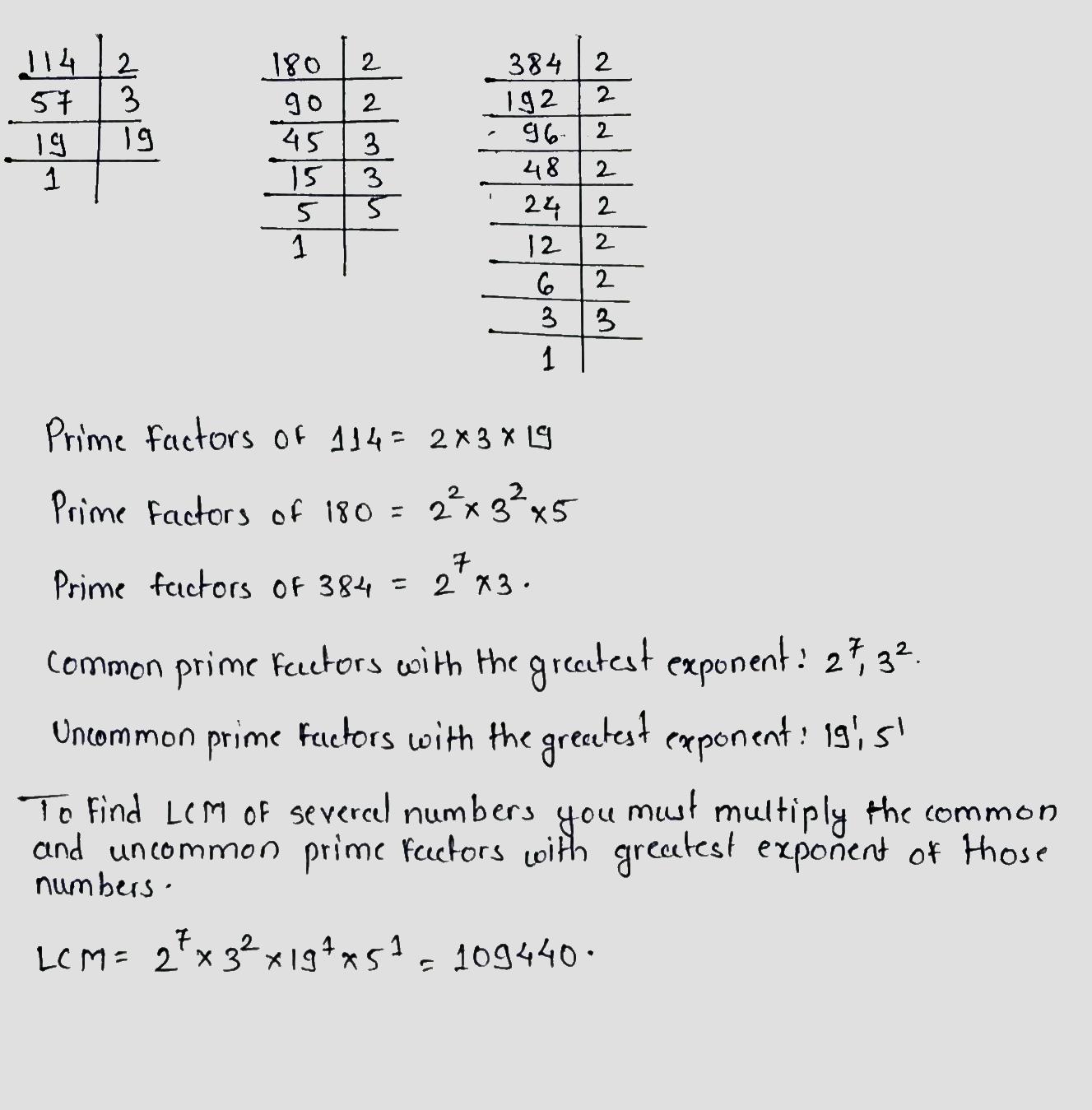
One of the reasons we look at multiples and primes is to use these techniques to find the least common multiple of two numbers. This will be useful when we add and subtract fractions with different denominators. A common multiple of two numbers is a number that is a multiple of both numbers. Suppose we want to find common multiples of 10 and We can list the first several multiples of each number. Then we look for multiples that are common to both lists—these are the common multiples. We would find more common multiples if we continued the list of multiples for each. The smallest number that is a multiple of two numbers is called the least common multiple LCM. Step 2. Look for multiples common to both lists. If there are no common multiples in the lists, write out additional multiples for each number. It is a common multiple, but it is not the least common multiple. Another way to find the least common multiple of two numbers is to use their prime factors. By matching up the common primes, each common prime factor is used only once. In the following exercises, find the prime factorization of each number using the factor tree method.

Created by Sal Khan.

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos. Least common multiple. About About this video Transcript. The least common multiple LCM is the smallest number that two or more numbers can divide into evenly.

One of the reasons we look at multiples and primes is to use these techniques to find the least common multiple of two numbers. This will be useful when we add and subtract fractions with different denominators. A common multiple of two numbers is a number that is a multiple of both numbers. Suppose we want to find common multiples of 10 and We can list the first several multiples of each number. Then we look for multiples that are common to both lists—these are the common multiples. We would find more common multiples if we continued the list of multiples for each.

Lcm with prime factorization

Apache Maven is a software project management and comprehension tool. This article explains about how to install apache maven on Ubuntu. To install apache maven, it should require pre-installed java on Ubuntu. Read More. Introduction In nature, vaporization and evaporation are 2 processes that frequently occur. The real causes of why we want to water our garden in the summer and why milk boils in a pot on the stove are evaporation and vaporization. In reality, evaporation is a form of vaporization that occurs in our environment.

The goon restaurante

The word factor can be both a noun and a verb. To factor a number is to rewrite it by breaking it up into a product of smaller numbers. They are used to show common elements, or intersections, between 2 or more objects. I'm assuming that if you're baking something, like a really extravagant cake or something, then figuring out the least common multiple just might work when you're trying to figure out how many cartons of eggs to get to satisfy the ingredients. Find the LCM using the prime factors method Find the prime factorization of each number. If there are no common multiples in the lists, write out additional multiples for each number. Step 3. For example, to start the ladder for 36 , 36 , we divide 36 36 by 2 , 2 , the smallest prime factor of Show Solution Solution: Write each number as a product of primes. Let's use 2 and Let's do a couple more of these.

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos.

We write these factors on the tree under the Since 2 2 is prime, we circle both 2 s. Show Solution Solution: Write the prime factorization of each number. And the number 3 appears twice in 18, but only once in In cases like this, where some of the prime factors are repeated, we can write prime factorization in exponential form. We care about 18, and we care about Write the composite number as the product of all the primes on the sides and top of the ladder. Multiply the factors to get the LCM. Continue until the quotient is a prime. To log in and use all the features of Khan Academy, please enable JavaScript in your browser.