Lcm of 40 48 and 45

In mathematics, the LCM of any two integers is the smallest value that is divisible by both of these integers. The LCM of 40, 48, and 45 is the smallest number that is a common multiple of these three numbers.

LCM of 40, 48 and 45 is The LCM of any two integers in mathematics is the value that is evenly divisible by the two values. LCM of 40, 48, and 45 is the smallest number among all common multiples of 40, 48, and The first few multiples of 40, 48, and 45 are 40, 80, , , There are 3 commonly used methods to find LCM of 40, 48, 45 — by listing multiples, by division method, and by prime factorization. Prime factorization, listing multiples, and division are the three most frequent methods for determining the LCM of 40, 48, and The answer to this question is

Lcm of 40 48 and 45

LCM of 40, 45 and 48 is equal to The comprehensive work provides more insight of how to find what is the lcm of 40, 45 and 48 by using prime factors and special division methods, and the example use case of mathematics and real world problems. Use in Mathematics: LCM of 40, 45 and 48 The below are some of the mathematical applications where lcm of 40, 45 and 48 can be used: to find the least number which is exactly divisible by 40, 45 and Use in Real-world Problems: 40, 45 and 48 lcm In the context of lcm real world problems, the lcm of 40, 45 and 48 helps to find the exact time when three similar and recurring with different time schedule happens together at the same time. For example, the real world problems involve lcm in situations to find at what time all the bells A, B and C toll together, if bell A tolls at 40 seconds, B tolls at 45 seconds and C tolls at 48 seconds repeatedly. The answer is that all bells A, B and C toll together at seconds for the first time, at seconds for the second time, at seconds for the third time and so on. Important Notes: 40, 45 and 48 lcm The below are the important notes to be remembered while solving the lcm of 40, 45 and The repeated and non-repeated prime factors of 40, 45 and 48 should be multiplied to find the least common multiple of 40, 45 and 48, when solving lcm by using prime factors method. The results of lcm of 40, 45 and 48 is identical even if we change the order of given numbers in the lcm calculation, it means the order of given numbers in the lcm calculation doesn't affect the results. The below solved example with step by step work shows how to find what is the lcm of 40, 45 and 48 by using either prime factors method and special division method. Solved example using prime factors method: What is the LCM of 40, 45 and 48?

Maths Program. Math worksheets and visual curriculum. Our Team.

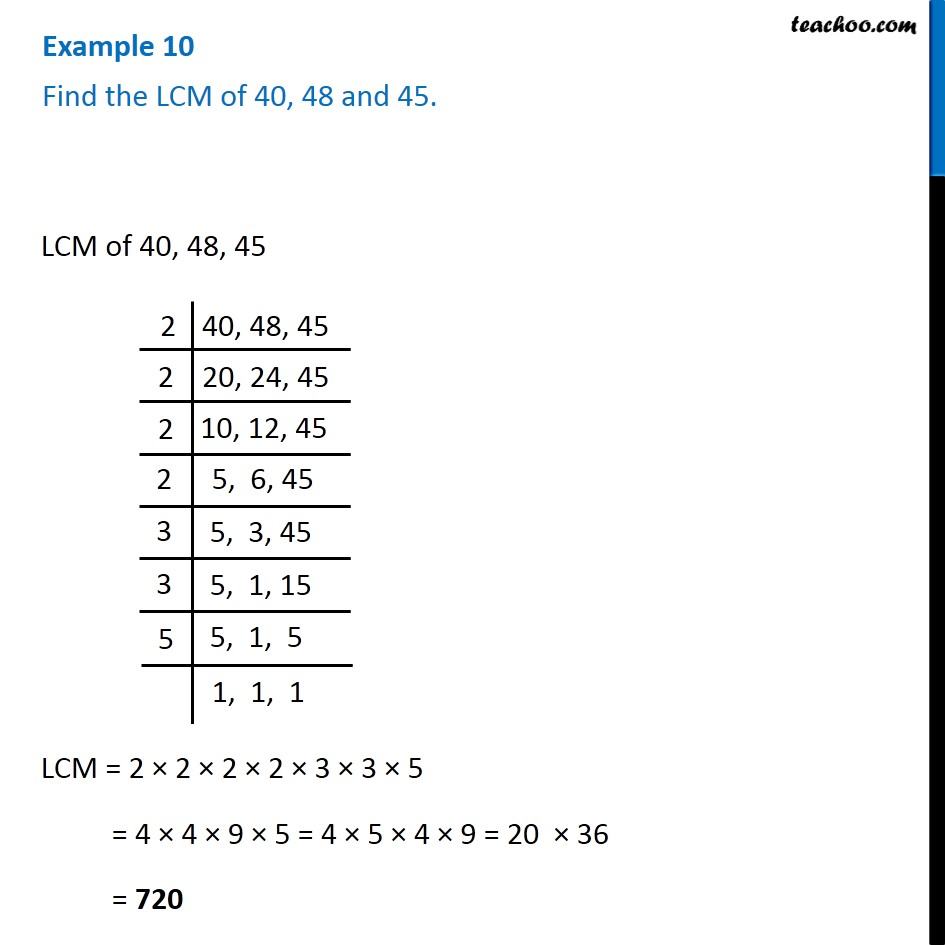
LCM of 40, 48, and 45 is the smallest number among all common multiples of 40, 48, and The first few multiples of 40, 48, and 45 are 40, 80, , , There are 3 commonly used methods to find LCM of 40, 48, 45 - by listing multiples, by division method, and by prime factorization. The LCM of three non-zero integers , a 40 , b 48 , and c 45 , is the smallest positive integer m that is divisible by a 40 , b 48 , and c 45 without any remainder. To calculate the LCM of 40, 48, and 45 by the division method, we will divide the numbers 40, 48, 45 by their prime factors preferably common.

LCM of 40, 48 and 45 is The LCM of any two integers in mathematics is the value that is evenly divisible by the two values. LCM of 40, 48, and 45 is the smallest number among all common multiples of 40, 48, and The first few multiples of 40, 48, and 45 are 40, 80, , , There are 3 commonly used methods to find LCM of 40, 48, 45 — by listing multiples, by division method, and by prime factorization. Prime factorization, listing multiples, and division are the three most frequent methods for determining the LCM of 40, 48, and

Lcm of 40 48 and 45

Please provide numbers separated by a comma "," and click the "Calculate" button to find the LCM. In mathematics, the least common multiple, also known as the lowest common multiple of two or more integers a and b , is the smallest positive integer that is divisible by both. It is commonly denoted as LCM a, b. There are multiple ways to find a least common multiple. The most basic is simply using a "brute force" method that lists out each integer's multiples. A more systematic way to find the LCM of some given integers is to use prime factorization. Prime factorization involves breaking down each of the numbers being compared into its product of prime numbers. The LCM is then determined by multiplying the highest power of each prime number together. Note that computing the LCM this way, while more efficient than using the "brute force" method, is still limited to smaller numbers. Refer to the example below for clarification on how to use prime factorization to determine the LCM:.

Warhammer 40000 titans

The value of LCM 40, 48, 45 will be the smallest number that is exactly divisible by 40, 48, and LCM of 40 48 and Explore math program. Multiplication Tables. The first few multiples of 40, 48, and 45 are 40, 80, , , Maths Games. Want to know more about this Super Coaching? For example, the real world problems involve lcm in situations to find at what time all the bells A, B and C toll together, if bell A tolls at 40 seconds, B tolls at 45 seconds and C tolls at 48 seconds repeatedly. Area Of A Circle Formula. Area Of Rectangle. Therefore, the LCM of 40, 48, and 45 is

The LCM calculator will determine the least common multiple of two to fifteen numbers for you - no need to fret!

Learn Lcm Of 40 48 And 45 with tutors mapped to your child's learning needs. Difference Between Correlation And Regression. The LCM of 40, 48 and 45 is Your Mobile number and Email id will not be published. The value of LCM 40, 48, 45 will be the smallest number that is exactly divisible by 40, 48, and The value of LCM 40, 48, 45 will be the smallest number that is exactly divisible by 40, 48, and Solved example using special division method: This special division method is the easiest way to understand the entire calculation of what is the lcm of 40, 45 and Commercial Maths. Solution: The value of LCM 40, 48, 45 will be the smallest number that is exactly divisible by 40, 48, and You may also be interested in: Lowest Common Multiple. For example, the real world problems involve lcm in situations to find at what time all the bells A, B and C toll together, if bell A tolls at 40 seconds, B tolls at 45 seconds and C tolls at 48 seconds repeatedly. List of Methods 3. The results of lcm of 40, 45 and 48 is identical even if we change the order of given numbers in the lcm calculation, it means the order of given numbers in the lcm calculation doesn't affect the results.