Lcm in division method
LCM by division method means finding the least common multiple of a given set of numbers by dividing all the given numbers by a common prime number. This is one of the easiest methods to find the LCM of numbers. Let us learn about the LCM by division method on this page, lcm in division method.
Both the methods are explained here with many examples. We have provided the prime factors of the given numbers, such as 24, 12, 30, , etc. The least or smallest common multiple of any two or more given natural numbers are termed as LCM. The largest or greatest factor common to any two or more given natural numbers is termed as HCF of given numbers. In the prime factorization method , given numbers are written as the product of prime factors. While in the division method, given numbers are divided by the least common factor and continue still remainder is zero.
Lcm in division method
For two integers a and b, denoted LCM a,b , the LCM is the smallest positive integer that is evenly divisible by both a and b. The LCM of two or more numbers is the smallest number that is evenly divisible by all numbers in the set. Find the LCM of a set of numbers with this calculator which also shows the steps and how to do the work. Input the numbers you want to find the LCM for. You can use commas or spaces to separate your numbers. But do not use commas within your numbers. For example, enter , and not 2,, 1, The LCM a,b is calculated by finding the prime factorization of both a and b. Use the same process for the LCM of more than 2 numbers. For example, for LCM 24, we find:. The cake method uses division to find the LCM of a set of numbers.
United States.
LCM by division is the process of finding the least common multiple of a given group of integers by dividing them by a common prime number. For this, we need to completely understand the prime factors of the given numbers. Prime factorization and listing the multiples are two additional techniques for calculating LCM. This technique of determining the LCM of numbers is one of the standards and provides a rapid result. We must understand the common multiplication tables and the prime numbers that can totally divide the provided values. To obtain the LCM using the common division method, we must first determine the prime factors of the provided integers. Step 1: Take the provided numbers and divide them by the least prime integer.
Both the methods are explained here with many examples. We have provided the prime factors of the given numbers, such as 24, 12, 30, , etc. The least or smallest common multiple of any two or more given natural numbers are termed as LCM. The largest or greatest factor common to any two or more given natural numbers is termed as HCF of given numbers. In the prime factorization method , given numbers are written as the product of prime factors. While in the division method, given numbers are divided by the least common factor and continue still remainder is zero. Note: Prime numbers are numbers which have only two factors i. Here, given natural numbers are written as the product of prime factors. The lowest common multiple will be the product of all prime factors with the highest degree power.
Lcm in division method
The HCF and LCM of a given set of numbers can be calculated using different methods like the division method and the prime factorization method. HCF refers to the Highest Common Factor HCF of two or more given numbers and it is the largest number that divides each of the given numbers without leaving any remainder. It is important to learn HCF and LCM in mathematics as it helps us to solve our day-to-day problems related to grouping and sharing. HCF is defined as the highest common factor present in two or more given numbers. For example, the HCF of 24 and 36 is 12, because 12 is the largest number which can divide both the numbers completely.
How to kill dough king
Ans: The number itself. Step 4: Keep dividing until the remaining is a prime number or one. Find the LCM enter two or more numbers. Coordinate System. Venn diagrams are drawn as overlapping circles. In order to find the LCM by division method, we use the same steps given above. In order to find the LCM by division method, we find a common prime number that divides the given numbers. So we divide 3 and 9 by 3 to get 1 and 3. Division of 24 and 15 by 3 will leave 8 and 5 as their remainders respectively. In this, the divisor is the prime number that would be divisible by the set of numbers given as the dividend. Step 2: Divide the numbers by 2 to get 12 and We continue the steps until 1 is left in the last row.
Least Common Multiple in maths is abbreviated as LCM and is used to find a number that is the smallest number that is divisible by two or more numbers perfectly.
The lowest common multiple will be the product of all prime factors with the highest degree power. The LCM of 60, 84, and is the least common multiple of 60, 84, and Then, we multiply the divisors written on the left to get the LCM of the given numbers. LCM by division method means finding the least common multiple of a given set of numbers by dividing all the given numbers by a common prime number. Post My Comment. We separate them with commas and find the prime numbers that can divide them. Step 2: Divide the numbers by 2 to get 12 and Multiply all divisors to get the HCF of given numbers. Multiples of , , , , , , , , , …. Here, given natural numbers are written as the product of prime factors. Division Table. Math worksheets and visual curriculum. Step 3: Now, we can multiply these prime numbers that are written on the left and then we can get the LCM of the numbers 62 and LCM of any prime number is. In using Venn diagrams to find the LCM, prime factors of each number, we call the groups, are distributed among overlapping circles to show the intersections of the groups.

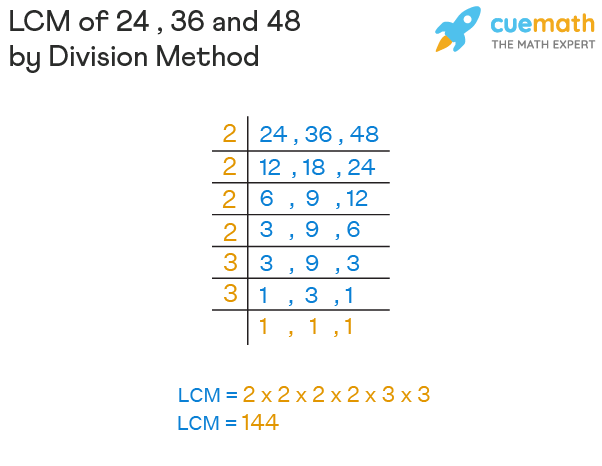
0 thoughts on “Lcm in division method”