Khan academy factorials

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Donate Log in Sign up Search for courses, skills, and videos. About About this video Transcript. Learn how to use permutations to solve problems involving ways to arrange things. Permutations involve using factorials to count all possible arrangements. This video also explores examples including arranging three people in three seats and five people in five seats. Want to join the conversation? Log in. Sort by: Top Voted. Emory Richardson. Posted 9 years ago. Okay, so this makes sense, but what's a good explanation for why we multiply instead of add, other than simply saying "because it gives us the right answer"? Downvote Button navigates to signup page.

Posted 8 months ago.

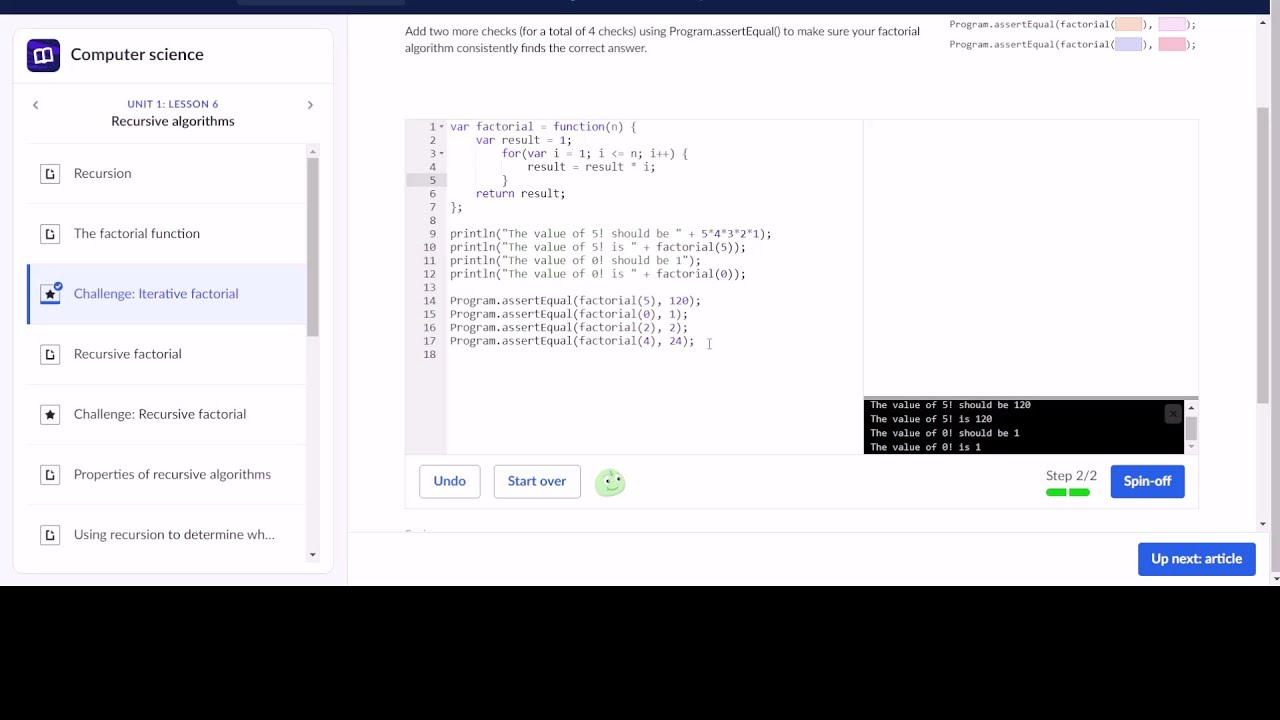
A factorial is a mathematical operation that you write like this: n! It represents the multiplication of all numbers between 1 and n. So if you were to have 3! Let's see how it works with some more examples. The factorial of a number is the multiplication of all the numbers between 1 and the number itself. It is written like this: n! So the factorial of 2 is 2! The factorial of 0 has value of 1, and the factorial of a number n is equal to the multiplication between the number n and the factorial of n Practically speaking, a factorial is the number of different permutations you can have with n items: 3 items can be arranged in exactly 6 different ways expressed as 3! You typically use a factorial when you have a problem related to the number of possible arrangements.

Khan academy factorials

The factorial function symbol:! It may seem funny that multiplying no numbers together results in 1, but let's follow the pattern backwards from, say, 4! One area they are used is in Combinations and Permutations. We had an example above, and here is a slightly different example:. The list is quite long, if the 7 people are called a,b,c,d,e,f and g then the list includes:. The formula is 7! So there are different ways that 7 people could come 1 st , 2 nd and 3 rd. Just shuffle a deck of cards and it is likely that you are the first person ever with that particular order. Note: it is called "Stirling's approximation" and is based on a simplifed version of the Gamma Function. Yes we can!

Colour blind glasses perth

Permutation formula Opens a modal. Comment Button navigates to signup page. Opens a modal. Gullara McInnes. Handshaking combinations Opens a modal. Log in. The number of branches at each node represents the number of choices available, and the number of nodes in the tree represents the number of steps or choices in the process. Posted 7 months ago. Posted 8 years ago. Zero factorial or 0! Bob Everton. Course challenge. It's just the product of the integers 1 through n. Direct link to K. The answer which we won't try to justify here turns out to be n!

How many different possibilities are there? Well, there's several ways to approach this. Let's look at an example: computing 5!. Posted 6 years ago. Log in. Recursive algorithms. We use 1. Switch your frame of reference - choose people for the chairs, and not chairs for the people. I was playing with formulae and accidentally came up with this Barbara DiLucchio. I think a good analogy for this is to think of a branching tree diagram. This is equal to n factorial. Comment Button navigates to signup page. Just wanted to help!

Khan academy factorials

Khan academy factorials

Khan academy factorials

Colour blind glasses perth

1 thoughts on “Khan academy factorials”

Leave a Reply Cancel reply