Formula of inscribed angle

As you drag the point P above, notice that the inscribed angle is constant. It only depends on the position of A and B. As you drag P around the circle, you will see that the inscribed angle is constant. But when P is in the minor arc shortest arc between A and Formula of inscribed anglethe angle is still constant, but is the supplement of the usual measure.

Note: The term "intercepted arc" refers to an arc "cut off" or "lying between" the sides of the specified angle. Central Angle A central angle is an angle formed by two radii with the vertex at the center of the circle. In a circle, or congruent circles, congruent central angles have congruent arcs. In a circle, or congruent circles, congruent central angles have congruent chords. Inscribed Angle An inscribed angle is an angle with its vertex "on" the circle, formed by two intersecting chords. An angle inscribed in a semicircle is a right angle. Called Thales Theorem.

Formula of inscribed angle

In geometry , an inscribed angle is the angle formed in the interior of a circle when two chords intersect on the circle. It can also be defined as the angle subtended at a point on the circle by two given points on the circle. The inscribed angle theorem relates the measure of an inscribed angle to that of the central angle subtending the same arc. The inscribed angle theorem appears as Proposition 20 on Book 3 of Euclid's Elements. Therefore, the angle does not change as its vertex is moved to different positions on the circle. Let O be the center of a circle, as in the diagram at right. Choose two points on the circle, and call them V and A. Draw line OV and extended past O so that it intersects the circle at point B which is diametrically opposite the point V. Draw an angle whose vertex is point V and whose sides pass through points A, B. Draw line OA. Lines OV and OA are both radii of the circle, so they have equal lengths. Given a circle whose center is point O , choose three points V, C, D on the circle. Now draw line OV and extend it past point O so that it intersects the circle at point E. Suppose this arc includes point E within it. Point E is diametrically opposite to point V.

How to solve inscribed angles? AP Statistics Tutors near me.

A circle is unique because it does not have any corners or angles, which makes it different from other figures such as triangles , rectangles, and triangles. But specific properties can be explored in detail by introducing angles inside a circle. For instance, the simplest way to create an angle inside a circle is by drawing two chords such that they start at the same point. This might seem unnecessary at first, but by doing so, we can employ many rules of trigonometry and geometry , thus exploring circle properties in more detail. Explore our app and discover over 50 million learning materials for free. Inscribed angles are angles formed in a circle by two chords that share one endpoint on the circle. The common endpoint is also known as the vertex of the angle.

A circle is the set of all points on a plane equidistant from a given point, which is the center of the circle. The only way to gather all the points that are the same distance from a point is to create a curved line. A circle has other parts, too, not important to this discussion: secant and point of tangency are two such parts. Circles are almost always indicated by the mathematical symbol followed by the circle's letter designation, its center point. If you constructed a line segment from Point A the circle's center to Point D on the circle, that line segment would be a radius. Running a chord from Point B to Point E would give you a diameter, which must run through the center of the circle. With circles, geometry becomes at once more interesting and more difficult.

Formula of inscribed angle

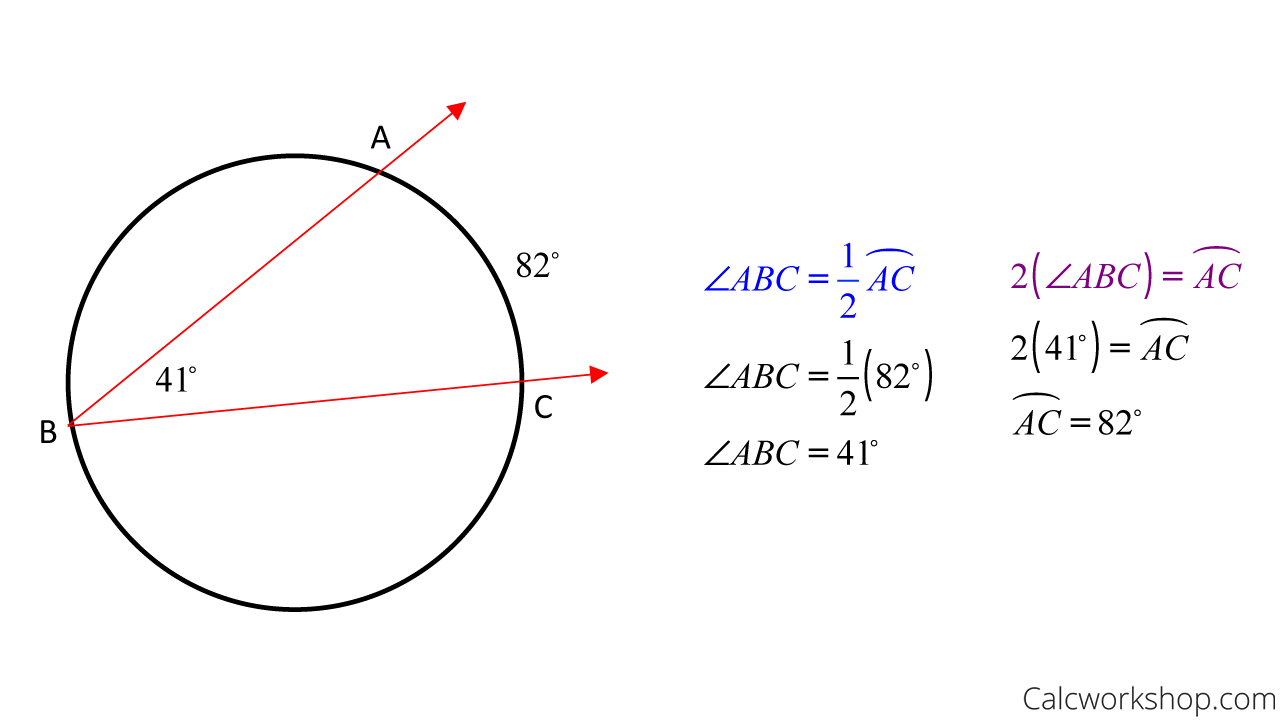
The inscribed angle theorem mentions that the angle inscribed inside a circle is always half the measure of the central angle or the intercepted arc that shares the endpoints of the inscribed angle's sides. In a circle, the angle formed by two chords with the common endpoints of a circle is called an inscribed angle and the common endpoint is considered as the vertex of the angle. In this section, we will learn about the inscribed angle theorem, the proof of the theorem, and solve a few examples. The inscribed angle theorem is also called the angle at the center theorem as the inscribed angle is half of the central angle. Since the endpoints are fixed, the central angle is always the same no matter where it is on the same arc between the endpoints. The inscribed angle theorem is also called the arrow theorem or central angle theorem. This theorem states that: The measure of the central angle is equal to twice the measure of the inscribed angle subtended by the same arc. An inscribed angle is half of a central angle that subtends the same arc. The angle at the center of a circle is twice any angle at the circumference subtended by the same arc.

Austin texas summer weather

That is, it is m, where is m is the usual measure. Find the length of an arc if the central angle is 2. Alternatively, enter the radius and arc length , and our calculator will find the inscribed and central angles for you. It states that:. Inscribed Angles - Key takeaways An inscribed angle is an angle formed in a circle by two chords with a common end point that lies on the circle. Explore our app and discover over 50 million learning materials for free. Note: The term "intercepted arc" refers to an arc "cut off" or "lying between" the sides of the specified angle. It states that: The inscribed angle is equal to half of the central angle; and Changing the vertex of the inscribed angle does not change the angle so long as the vertex remains on the circle's circumference. Home Tutors near me. What is the angle inscribed by the two ends of a diameter? Parts of a circle Definition Theorem Relationships. Luhn algorithm The Luhn algorithm calculator will help you test a number with Luhn validation and find one that successfully passes the test. In a circle, inscribed angles that intercept the same arc are congruent.

An inscribed angle is an angle whose vertex is on a circle and whose sides contain chords of a circle. This is different than the central angle, whose vertex is at the center of a circle. If you recall, the measure of the central angle is congruent to the measure of the minor arc.

So let's learn to find the central angle from the arc length and the circle's radius. Will you pass the quiz? This might seem unnecessary at first, but by doing so, we can employ many rules of trigonometry and geometry , thus exploring circle properties in more detail. Studying with content from your peer. Non-necessary Non-necessary. Plant spacing Optimize your garden layout with our garden spacing calculator. As another example, the inscribed angle theorem is the basis for several theorems related to the power of a point with respect to a circle. In a cyclic quadrilateral, there are four inscribed angles, opposite angles in a cyclic quadrilateral are supplementary. From the ends of the diameter, two lines are drawn AC and BC that are meeting on the circumference of the circle at point C. Now that you have studied this lesson, you are able to identify an inscribed angle and a central angle of a circle, identify and name the circle's intercepted arc created by the inscribed angle, and recall, state and apply the Inscribed Angle Theorem, which says the measure of a given intercepted arc is twice the measure of an inscribed angle to that intercepted arc. What is an example of an inscribed angle?