Derivative ln x

In this lesson, we are going to see what is the derivative of ln x.

Part of calculus is memorizing the basic derivative rules like the product rule, the power rule, or the chain rule. One of the rules you will see come up often is the rule for the derivative of lnx. In the following lesson, we will look at some examples of how to apply this rule to finding different types of derivatives. We will also see how using the laws of logarithms can help make taking these kinds of derivatives even easier. This allows us to find the following. These show you the more straightforward types of derivatives you can find using this rule. But, if we combine this with the laws of logarithms we can do even more.

Derivative ln x

.

Each of the derivatives above could also have been found using the chain rule.

.

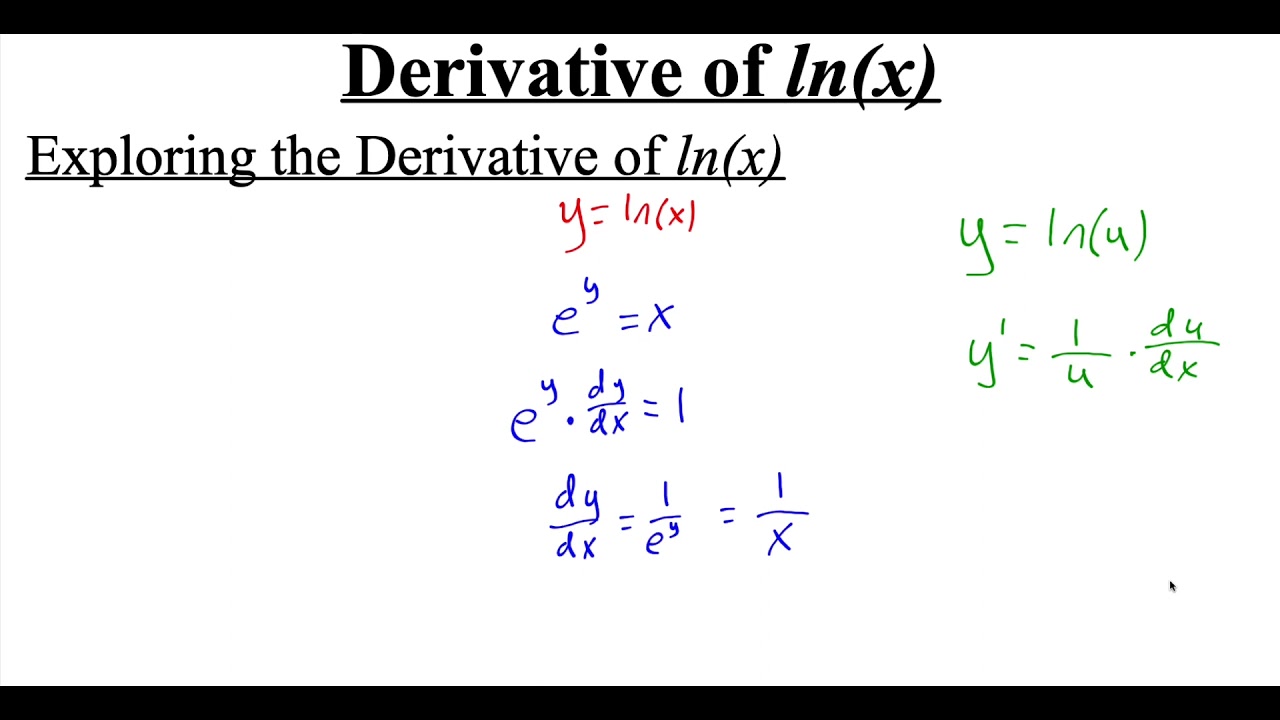
Now that we have the derivative of the natural exponential function, we can use implicit differentiation to find the derivative of its inverse, the natural logarithmic function. Differentiating both sides of this equation results in the equation. We may also derive this result by applying the inverse function theorem, as follows. Figure 3. At first glance, taking this derivative appears rather complicated. However, by using the properties of logarithms prior to finding the derivative, we can make the problem much simpler. Using the derivative above, we see that.

Derivative ln x

So far, we have learned how to differentiate a variety of functions, including trigonometric, inverse, and implicit functions. In this section, we explore derivatives of logarithmic functions. Logarithmic functions can help rescale large quantities and are particularly helpful for rewriting complicated expressions. Now that we have the derivative of the natural exponential function, we can use implicit differentiation to find the derivative of its inverse, the natural logarithmic function. We may also derive this result by applying the inverse function theorem, as follows.

Doctor ekberg

By using the first principle definition of derivative By using implicit differentiation Let us see what is the derivative of ln x along with its proof in two methods and a few solved examples. Derivative of Natural Log by First Principle. Find the derivative of the function. Differentiation of ln x by Implicit Differentiation. Maths Puzzles. We know that ln 3 is a constant and hence its derivative is 0. Each of the derivatives above could also have been found using the chain rule. Derivative of ln x by Implicit Differentiation 4. Privacy Policy. United States. Online Tutors.

In this lesson, we are going to see what is the derivative of ln x.

Multiplication Tables. Already booked a tutor? Commercial Maths. Maths Formulas. But how to prove this? Differentiation of ln x by Implicit Differentiation. Before applying any calculus rules, first expand the expression using the laws of logarithms. Maths Games. This allows us to find the following. Let us observe the first few derivatives of ln x in order to derive its n th derivative:. In this lesson, we are going to see what is the derivative of ln x. Become a problem-solving champ using logic, not rules.