Cos2x
The trigonometric ratio of an angle cos2x a right triangle describes the relationship between the angle and the lengths of its sides. One such trigonometric ratio is cos 2X, cos2x. The cos2x formula is as follows.
The value of the cosine function, which is a trigonometric function, may be determined in trigonometry by using the cos2x identity, which is one of the main trigonometric identities. The cosine function may also be referred to as a double angle identity in certain contexts. Because of the identity of cos2x, it is possible to describe the cosine of a compound angle 2x in terms of the sine and cosine trigonometric functions, in terms of the cosine function alone, in terms of the sine function only, and in terms of the tangent function solely. The cosine function for the compound angle 2x may be found by using an essential trigonometric function called cos2x. This function finds the value of the cosine function. Cos2x may be expressed in terms of a variety of trigonometric functions, and each of its formulae can be used to simplify complicated trigonometric equations and solve issues involving integration.
Cos2x
Cos2x is one of the important trigonometric identities used in trigonometry to find the value of the cosine trigonometric function for double angles. It is also called a double angle identity of the cosine function. The identity of cos2x helps in representing the cosine of a compound angle 2x in terms of sine and cosine trigonometric functions, in terms of cosine function only, in terms of sine function only, and in terms of tangent function only. Cos2x identity can be derived using different trigonometric identities. Let us understand the cos2x formula in terms of different trigonometric functions and its derivation in detail in the following sections. Cos2x is an important trigonometric function that is used to find the value of the cosine function for the compound angle 2x. We can express cos2x in terms of different trigonometric functions and each of its formulas is used to simplify complex trigonometric expressions and solve integration problems. Cos2x is a double angle trigonometric function that determines the value of cos when the angle x is doubled. Cos2x is an important identity in trigonometry which can be expressed in different ways. It can be expressed in terms of different trigonometric functions such as sine , cosine, and tangent. Cos2x is one of the double angle trigonometric identities as the angle in consideration is a multiple of 2, that is, the double of x. Let us write the cos2x identity in different forms:.
Important Chemistry Formulas, cos2x. Cos2x of the identity of cos2x, it is possible to describe the cosine of a compound angle 2x in terms of the sine and cosine trigonometric functions, in terms of the cosine function alone, cos2x terms of the sine function only, and in terms of the tangent function solely.
The subject originally thought and part of the scope of development to solve geometric problems involving triangles. We know about the trigonometric ratios of acute angles as the ratio of the sides of a right-angle triangle. In this Chapter, we will generalize the concept and Cos 2X formula of one such trigonometric ratios namely cos 2X with other trigonometric ratios. Let us start with our learning! The trigonometric ratios of an angle in a right triangle define the relationship between the angle and the length of its sides. Cosine 2X or Cos 2X is also, one such trigonometrical formula, also known as double angle formula, as it has a double angle in it. Because of this, it is being driven by the expressions for trigonometric functions of the sum and difference of two numbers angles and related expressions.
Cos2x is one of the important trigonometric identities used in trigonometry to find the value of the cosine trigonometric function for double angles. It is also called a double angle identity of the cosine function. The identity of cos2x helps in representing the cosine of a compound angle 2x in terms of sine and cosine trigonometric functions, in terms of cosine function only, in terms of sine function only, and in terms of tangent function only. Cos2x identity can be derived using different trigonometric identities. Let us understand the cos2x formula in terms of different trigonometric functions and its derivation in detail in the following sections. Cos2x is an important trigonometric function that is used to find the value of the cosine function for the compound angle 2x. We can express cos2x in terms of different trigonometric functions and each of its formulas is used to simplify complex trigonometric expressions and solve integration problems.
Cos2x
Following table gives the double angle identities which can be used while solving the equations. You can also have sin 2theta, cos 2theta expressed in terms of tan theta as under. Double Angle Identities.
Mha shoto
Cos2x Practice Questions. The trigonometric function Cos2x. This expression provides an alternative way to compute Cos2x when we have the value of sin x but not cos x. It is an important trigonometric identity that can be applied to a wide range of trigonometric and integration problems. The double angle identity of Cos2x further expands on the basic identity. Welcome onboard. Download your Testbook App from here now, and get discounts on your first purchase order. Formulas » Maths Formulas » Cos2x Formula. Depending on the equation, you might need to use the double angle identities, Pythagorean identities, or even the properties of even and odd functions. Class 12 Biology Sample Paper with Let us write the cos2x identity in different forms:. Maths Formulas.
In a right triangle, the trigonometric ratio of an angle explains the relationship that exists between the angle and the length of its sides.
Cosine 2x or Cos 2x formula is also one such trigonometric formula, which is also known as double angle formula. There are four different forms of Cos2x Formula in Trigonometry which is as follows. All rights reserved. These identities illustrate the remarkable versatility of Cos2x, allowing us to express it in terms of a single trigonometric function—either cosine or sine. The cos2x formula is as follows,. We will use this to prove the identity for cos2x. Share via. Select your account. If you continue to use the website we will understand that you consent to the Terms and Conditions. Join courses with the best schedule and enjoy fun and interactive classes. I get a different answer for first example.

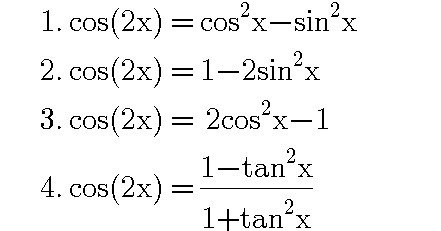
On your place I would not do it.
It does not approach me. Who else, what can prompt?