Cos 2 x sin 2 x

A trigonometric identity, sin 2x cos 2x, is required to resolve a number of trigonometric problems. This article includes the integral and derivative of sin2x and cos2x in addition to their simplified values. The ratio between the opposing side and the hypotenuse of an angle is represented by the sin function.

Sign in to comment. Sign in to answer this question. Unable to complete the action because of changes made to the page. Reload the page to see its updated state. Choose a web site to get translated content where available and see local events and offers. Based on your location, we recommend that you select:.

Cos 2 x sin 2 x

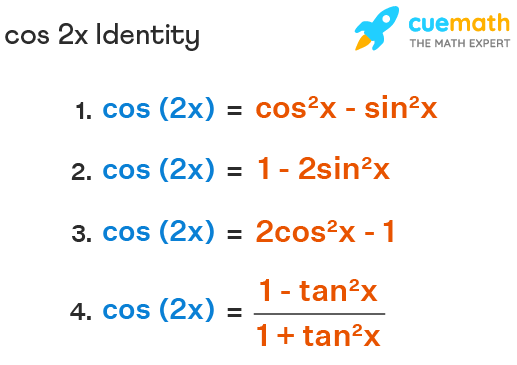
Cos2x is one of the important trigonometric identities used in trigonometry to find the value of the cosine trigonometric function for double angles. It is also called a double angle identity of the cosine function. The identity of cos2x helps in representing the cosine of a compound angle 2x in terms of sine and cosine trigonometric functions, in terms of cosine function only, in terms of sine function only, and in terms of tangent function only. Cos2x identity can be derived using different trigonometric identities. Let us understand the cos2x formula in terms of different trigonometric functions and its derivation in detail in the following sections. Cos2x is an important trigonometric function that is used to find the value of the cosine function for the compound angle 2x. We can express cos2x in terms of different trigonometric functions and each of its formulas is used to simplify complex trigonometric expressions and solve integration problems. Cos2x is a double angle trigonometric function that determines the value of cos when the angle x is doubled. Cos2x is an important identity in trigonometry which can be expressed in different ways. It can be expressed in terms of different trigonometric functions such as sine , cosine, and tangent. Cos2x is one of the double angle trigonometric identities as the angle in consideration is a multiple of 2, that is, the double of x. Let us write the cos2x identity in different forms:.

Explore math program.

This maybe is not a very nice proof for the identities themselves for a trigonometry student, but I find it a very useful way to derive the formula if you can't remember it. Since the imaginary parts on the left must equal the imaginary parts on the right and the same for the real, we can deduce the following relationships:. And with that, we've proved both the double angle identities for sin and cos at the same time. In fact, using complex number results to derive trigonometric identities is a quite powerful technique. You can for example prove the angle sum and difference formulas with just a few lines using Euler's identity.

Cos2x is one of the important trigonometric identities used in trigonometry to find the value of the cosine trigonometric function for double angles. It is also called a double angle identity of the cosine function. The identity of cos2x helps in representing the cosine of a compound angle 2x in terms of sine and cosine trigonometric functions, in terms of cosine function only, in terms of sine function only, and in terms of tangent function only. Cos2x identity can be derived using different trigonometric identities. Let us understand the cos2x formula in terms of different trigonometric functions and its derivation in detail in the following sections.

Cos 2 x sin 2 x

In mathematics, an "identity" is an equation which is always true, regardless of the specific value of a given variable. Need a custom math course? K12 College Test Prep.

Fishing bundle stardew

We can express cos2x in terms of different trigonometric functions and each of its formulas is used to simplify complex trigonometric expressions and solve integration problems. Support Answers MathWorks. Related questions What does it mean to prove a trigonometric identity? Cos2x Cos2x is one of the important trigonometric identities used in trigonometry to find the value of the cosine trigonometric function for double angles. In fact, using complex number results to derive trigonometric identities is a quite powerful technique. Maths Program. Based on your location, we recommend that you select:. A function's derivative is provided at each point in the domain of the original function for which the derivative is defined by the derivative function. You will be using all of these identities, or nearly so, for proving other trig identities and for solving trig equations. See Also.

.

Let us consider an example to understand the application of cos2x formula. Several sin2x formulas can be checked using fundamental trigonometric formulas. Cancel Copy to Clipboard. This article includes the integral and derivative of sin2x and cos2x in addition to their simplified values. Learn Practice Download. Solved example Problem: 1 Find the general solutions to the identified equations. Logically, mathematical identities are tautologies; that is, they are expressions which restate the same expression in a different way. Cos2x is one of the important trigonometric identities used in trigonometry to find the value of the cosine trigonometric function for double angles. It is also called a double angle identity of the cosine function. You will be using all of these identities, or nearly so, for proving other trig identities and for solving trig equations. Bruno Luong on 17 Nov