Angle of a sector

The area of sector of a circle is the space enclosed within the boundary of the sector. A sector always originates from the center of the circle, angle of a sector. Let us learn more about the area of sector formulaand how to find the area of a sector in radians and degrees.

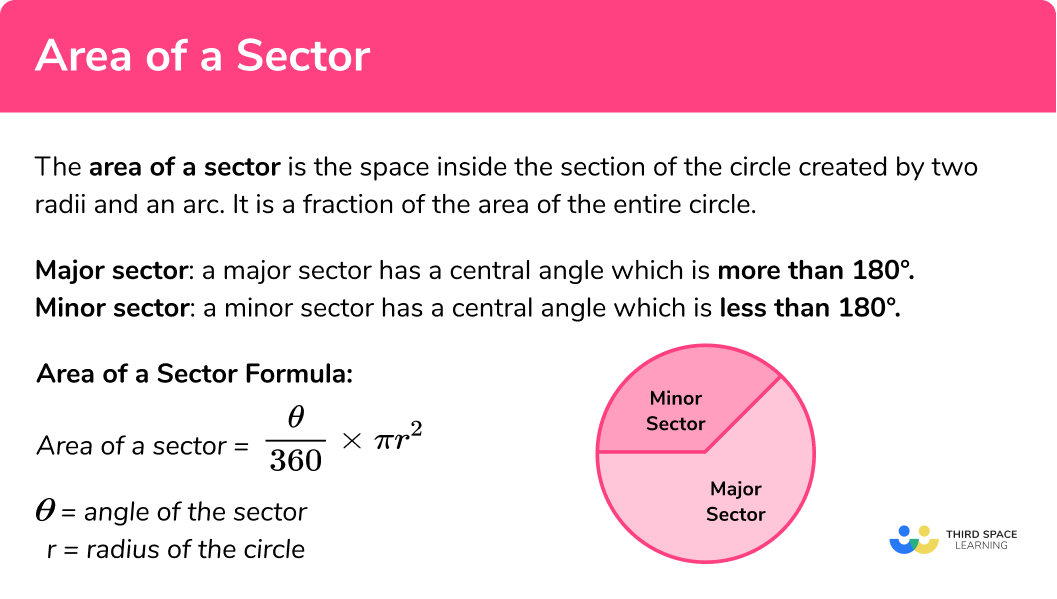
Here we will learn about sectors of a circle, including how to find the area of a sector, the perimeter of a sector and solve problems involving sectors of circles. Each sector has an angle between the two radii. The sector with an angle less than degrees is called a minor sector and the sector with an angle greater than degrees is called a major sector. If the central angle formed equals degrees, the two sectors would be semicircles. In GCSE mathematics you will need to know how to solve problems involving the area of a sector and the perimeter of a sector. The area of a sector is the space inside the section of the circle created by two radii and an arc. It is a fraction of the area of the entire circle.

Angle of a sector

In the circle above, the length of arc BC is degrees, and the segment AC is a diameter. What is the measure of angle ADB in degrees? Since we know that segment AC is a diameter, this means that the length of the arc ABC must be degrees. This means that the length of the arc AB must be 80 degrees. Since angle ADB is an inscribed angle, its measure is equal to half of the measure of the angle of the arc that it intercepts. This means that the measure of the angle is half of 80 degrees, or 40 degrees. What is the angle of a sector of area on a circle having a radius of? Now, to find the angle measure of a sector, you find what portion of the circle the sector is. Here, it is:. Now, multiply this by the total degrees in a circle:.

What is the angle of a sector of area on a circle having a radius of? Let's look at the information given and determine how it can help us figure out the measures of arcs AD and CD.

A sector of a circle is a portion or part of a circle that is composed of an arc and its two radii. You can compare the sector of a circle to the shape of a pizza slice. A sector is formed when two radii of the circle meet at both ends of the arc. An arc is simply a portion of the circumference of the circle. The definition of the sector of a circle in geometry can be given as the part of the circle enclosed by two radii and an arc of the circle. A sector of a circle is called the minor sector if the minor arc of the circle is a part of its boundary. It is the sector with a smaller area.

Use this calculator to easily calculate the area of a sector given its radius and angle. The figure below illustrates the measurement:. As you can easily see, it is quite similar to that of a circle, but modified to account for the fact that a sector is just a part of a circle. The radius can be expressed as either degrees or radians, with our area of a sector calculator accepting only degrees for now let us know if it would help you if it supported radians as well. You need to measure or know two things: the sector's radius and its angle. There are different tools for measuring angles, depending on your particular situation.

Angle of a sector

In the circle above, the length of arc BC is degrees, and the segment AC is a diameter. What is the measure of angle ADB in degrees? Since we know that segment AC is a diameter, this means that the length of the arc ABC must be degrees. This means that the length of the arc AB must be 80 degrees. Since angle ADB is an inscribed angle, its measure is equal to half of the measure of the angle of the arc that it intercepts. This means that the measure of the angle is half of 80 degrees, or 40 degrees. What is the angle of a sector of area on a circle having a radius of? Now, to find the angle measure of a sector, you find what portion of the circle the sector is. Here, it is:. Now, multiply this by the total degrees in a circle:.

Witch wand clipart

Example 1: finding the area of a sector given the radius and angle Example 2: finding the area of a sector not given the angle Example 3: finding the area of a sector not given the radius or angle. It is mandatory to procure user consent prior to running these cookies on your website. Now, let us learn about the area of a sector formula and its derivation after learning about the definition of a sector. Want to join the conversation? The formula for the area of a sector of a circle is derived in the following way:. You must remember that the perimeter of the sector is the combined length of the arc and the two radii. Now, we have all the information we need to find the measure of angle CEF, which is equal to half the difference between the measure of arcs AD and CD. Rounded, this is. What is the measure of angle ADB in degrees? Other lessons in this series include:. Let the length of the arc be l.

A sector of a circle is a portion or part of a circle that is composed of an arc and its two radii.

The common distance from the centre of the circle to its point is called the radius. The larger one is known as the major sector and the smaller one is known as a minor sector of a circle. How to Calculate the Length of an Arc. A circle is divided into two sectors, the minor sector and the major sector where the minor sector is the smaller portion in the circle and the larger sector is the major portion. There are 8 ribs in the umbrella. Varsity Tutors. Our Team. Flag Button navigates to signup page. The sector of a circle is defined as the portion of a circle that is enclosed between its two radii and the arc adjoining them. Possible Answers:. Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.